
Analytical standard calculator manual#
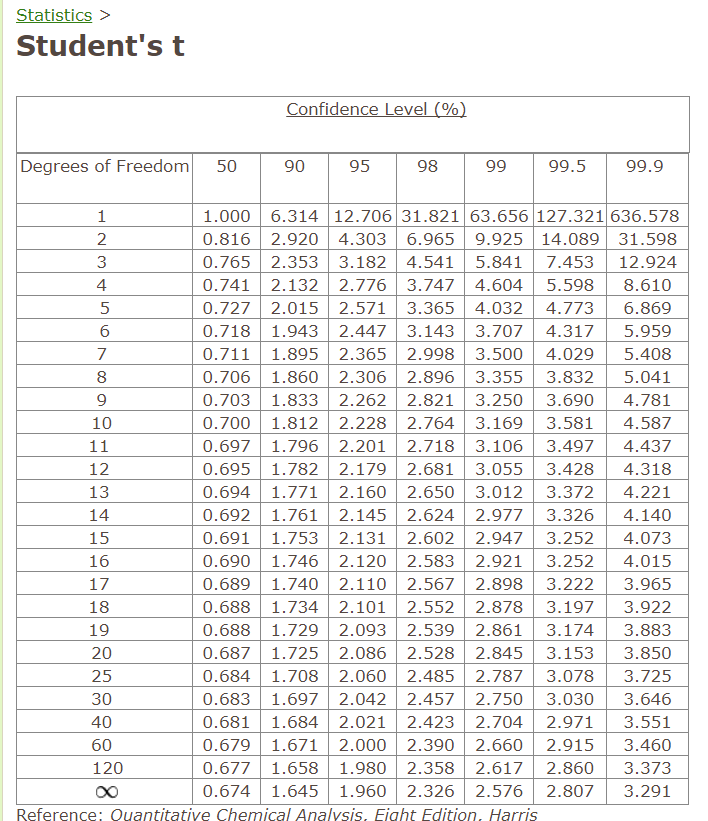
While it is beyond the scope of this manual to explain the statistical significance of standard deviation, the formula for calculating it is quite straight forward and is given below A common way to express precision is through the parameter called the standard deviation. The precision is a measure of how closely grouped the individual values are around the average value. The general expression for reporting the relative error uses the absolute value of the true value in the denominator so that the correct sign in the final relative value is obtained. Since measured or true values may sometimes be negative (such as a freezing point with a negative Celsius value), the general expression for reporting absolute error is (experimental value – true value), where the values are written with the appropriate negative or positive sign. Thus the relative error in this measurement is Parts per thousand is similar to percent, since percent is just a relative measurement in terms of parts per hundred. A common way of reporting errors is to express the error relative to the true value, either in terms of percent or parts per thousand (ppt). If the true density of this sample is actually 0.1100 g L−1, then the error is The average density is then 0.1095 g L−1. Because each of the volume and mass measurements have some uncertainty due to estimation of the last digit, the reported densities could have values of 0.1093, 0.1102 and 0.1089 g L−1.
To illustrate these two concepts, consider an experiment in which the density of the nitrogen gas sample mentioned in the previous section (Rounding Off) was determined three times. In reporting experimental results two important issues are: 1) how close is the experimentally reported average to the true value and 2) how reproducible or closely grouped was the data used in determining the average? The first issue deals with the concept of accuracy while the second deals with precision. This is the reason that you are generally asked to perform measurements in triplicate and report the average value. However, even as few as three measurements can greatly reduce the impact of random errors.While a large number of experimental measurements effectively reduces (but never eliminates) the uncertainty due to random errors, it is usually impractical in terms of time and money to make a large number of measurements.Since random errors will be statistically distributed in both the high and low direction to an equal extent, making several measurements and reporting the average value tends to reduce the influence of random error when compared to just a single measurement.If the uncertainty is due to random errors in the measuring process, then a set of replicate measurements will have several different values depending on the estimate that is made for each measurement.As mentioned in the discussion of significant figures, there is always some uncertainty in a measured quantity because the last significant figure is based upon estimation.Guideline 69 )/RD/Type/Annot/Popup 832 0 R/AP>endobj832 0 objendobj833 0 obj/Type/XObject/BBox/FormType 1>streamĮndstreamendobj834 0 obj/Type/XObject/BBox/FormType 1>streamĮndstreamendobj835 0 obj/Type/XObject/BBox/FormType 1>streamĪcrobat Distiller 6.0 (Windows) GUIDELINES FOR THE VALIDATION OF ANALYTICAL METHODS FOR ACTIVE CONSTITUENT, AGRICULTURAL AND VETERINARY CHEMICAL PRODUCTS


 0 kommentar(er)
0 kommentar(er)
